G4 Solution
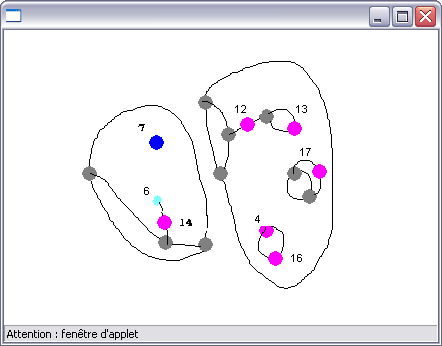 |
| The G4 Puzzle |
So the only winning move is to convert the G4 to a G3 as G3+G3=G0 (genus sequence starts by 0^0 so winning also for misère Sprouts).
Let's see four options of value G0, G1, G2, G3 of the right biosphere:
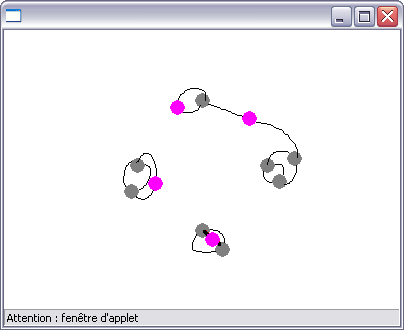 | 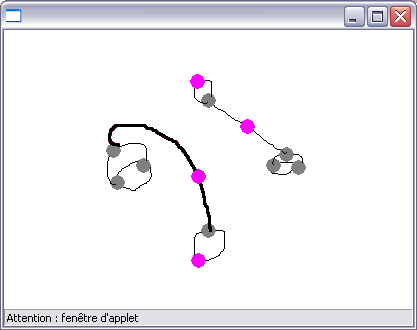 |
| G0 (Grundy number = 0) | G1 (Grundy number = 1) |
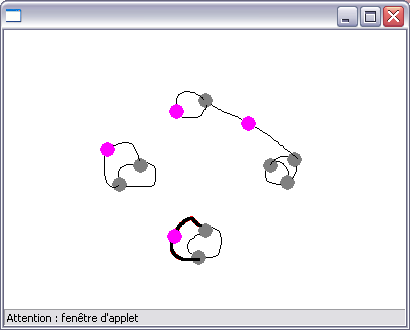 |  |
| G2 (Grundy number = 2) | G3 (Grundy number = 3) |
there are in fact three ways to get a G3, by
connecting together two of the top
three cannibals, leaving the airbag
alone, each one gives a valid similar solution.
Now let see six possible
false tries :
- Playing a G2 in the G4 by 4(18@12)16 gives G3+G2=G1 (1^1), a possible answer is to convert the
G3 into a G2 by 6(19)14 : G2+G2=G0
- Playing a G1 in the G4 by 4(18)17 gives
G3+G1=G2 (2^2), a possible answer is to
convert the G3 into a G1 in normal Sprouts by 6(19)6[7] and to convert the G3
into a G0 in misere Sprouts by 6(19)6 as 0^1+1^0=1^0 winning misère
position
- Playing a G0 in the G4 by 4(18)16 gives G3+G0=G3 (3^3), a possible answer is to convert the
G3 into a G0 in normal Sprouts by 6(19)6 and into a G1 in misère Sprouts by
6(19)6[7] as 1^0+0^1=1^0 winning misère position
- Playing a G0
in the G3 by 6(18)6 gives G0+G4=G4 (4^4), a possible answer is to convert the
G4 into a G0 in normal Sprouts by 4(19)16
and into a G1 in misère Sprouts by 4(19)17
- Playing a G1 in the G3 by
6(18)6[7] gives G1+G4=G5 (5^5), a possible answer is to convert the
G4 into a G1 in normal Sprouts by 4(19)17
and into a G0 in misère Sprouts by 4(19)16
- Playing a G2 in the G3 by 6(18)14 gives G2+G4=G6 (6^6), a possible answer is to convert the
G4 into a G2 by 4(19@12)16 both in normal
or misere Sprouts as 2^2+2^2=0^0 a winning position for both
variants.